Question

Find the length of the curve yequalsthree fourths x Superscript 4 divided by 3 Baseline minus three eighths x Superscript 2 divided by 3 Baseline plus 5 for 1less than or equalsxless than or equals27.

Answer 1

Explanation:

Consider the equation of the curve,

`y(x)=(3)/(4)x^{(4)/(3)}-(3)/(8)x^{(2)/(3)}+5:1\leq x \leq 27`

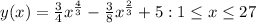
Find the arc length directly as follows:

Arc length of the curve:

if
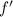`f'` is continous on [a,b], then the length of the curve
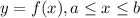`y=f(x),a\leq x \leq b` is

`L=\int\limits^b_a {\sqrt{1+[(dy)/(dx) ]^2} } \, dx`

Differentiate the function with respect to x,

`(dy)/(dx)= (d)/(dx)[(3)/(4)^{(4)/(3) }-(3)/(8)^{(2)/(3) }+5]\\\\=(d)/(dx)[(3)/(4)^{(4)/(3) }]-(d)/(dx)[(3)/(8)^{(2)/(3) }]+(d)/(dx)(5)\\\\=(3)/(4) (d)/(dx)[x^{(4)/(3)}] -(3)/(8) (d)/(dx)[x^{(2)/(3)}]+(d)/(dx)(5)\\ \\=(3)/(4)[(4)/(3)x^{(1)/(3) } ] +(3)/(8)[(2)/(3)x^{(1)/(3) } ]+0\\ \\=x^{(1)/(3)}-(1)/(4)x^{(1)/(3)}`use
`(d)/(dx)[x^n]=nx^(n-1)`

That is,

`((dy)/(dx) )^2=(x^{(1)/(3) }-(1)/(4)x^(1)/(3) )^2\\\\=x^{(1)/(3) }+(1)/(16)x^{(1)/(3) }-(1)/(2)\\\\\sqrt{1+[(dy)/(dx) ]^2}=\sqrt{1+x^{(1)/(3) }+(1)/(16)x^{(1)/(3) }-(1)/(2)} \\\\ =\sqrt{x^{(1)/(3) }+(1)/(16)x^{(1)/(3) }+(1)/(2)}`

since
`[x^{(1)/(3)}+(1)/(4)x^{-(1)/(3)}]^2=x^{(1)/(3)}+(1)/(16)x^{-(2)/(3)}+(1)/(2)`

`=[x^{(1)/(3)}+(1)/(4)x^{-(1)/(3)}]^2\\\\=x^{(1)/(3)}+(1)/(4)x^{-(1)/(3)}`

substitute this value in arc length formula to get,

`L=\int\limits^(27)_1 {[x^{(1)/(3)}]+(1)/(4)x^{-(1)/(3)}} \, dx \\\\=\int\limits^(27)_1 {[x^{(1)/(3)}]\, dx + (1)/(4)\int\limits^(27)_1 [x^{-(1)/(3)}}] \, dx\\\\=(3)/(4) [x^{(1)/(3)}]\limits^(27)_1 + (1)/(4) [(3)/(2)x^{(1)/(3)}]\limits^(27)_1`

use
`\int {x^n}=(x^(n+1))/(n+1)`

`=(3)/(4) [27^{(4)/(3) }-1]+(3)/(8) [27^{(2)/(3)}-1]\\\\=63`

therefore the length of the curves is L=63