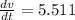Answer:

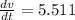
Step-by-step explanation:
The acceleration field is obtained by deriving the components in function of the time. That is to say:

Where
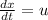 and
and
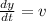 .
.
The velocity components at given point are, respectively:
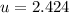
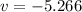
Lastly, the acceleration components are found: