Answer:
(a) The expected cash flow from Palmer Heights is $30,000 and from Crenshaw Village is $25,000.
(b) The coefficient of variation for Palmer Heights is 43.47% and for Crenshaw Village is 31%.
Explanation:
The formula to compute the expected value of a discrete probability distribution is:
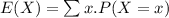
The formula to compute the variance of a discrete probability distribution is:
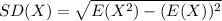
The formula to compute the coefficient of variation is:
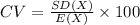
Denote:
X = Palmer Heights
Y = Crenshaw Village
(a)
Compute the expected cash flow from Palmer Heights as follows:
![E(X)=\sum x.P(X=x)\\=[(10*0.1)+(15*0.2)+(30*0.4)+(45*0.2)+(50*0.1)]\\=30](https://img.qammunity.org/2021/formulas/mathematics/college/tqo8wd7zexmgv10wy1kz5u35saxy1ezk57.png)
Compute the expected cash flow from Crenshaw Village as follows:
![E(Y)=\sum y.P(Y=y)\\=[(15*0.2)+(20*0.3)+(30*0.4)+(40*0.1)]\\=25](https://img.qammunity.org/2021/formulas/mathematics/college/mrufnrbndlwisp2uq2mj2s1m9r9kkr4fyh.png)
Thus, the expected cash flow from Palmer Heights is $30,000 and from Crenshaw Village is $25,000.
(b)
Compute the standard deviation of cash flow from each apartment as follows:
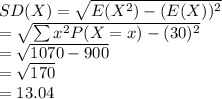
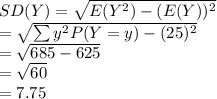
Compute the coefficient of variation for Palmer Heights as follows:
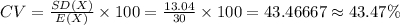
Compute the coefficient of variation for Crenshaw Village as follows:
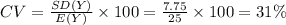
Thus, the coefficient of variation for Palmer Heights is 43.47% and for Crenshaw Village is 31%.