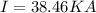Question

Consider an InSb NW with ballistic mean free path of 150nm. Calculate the current through a 250nm long InSb NW when a 100mV bias is applied. Assume that valley degeneracy 1.

Answer 1

`I =38.46KA`

Step-by-step explanation:

Given parameters include:

V = 100mV = 100 × 10⁻³ V

mean free path(W) = 150nm = 150 × 10⁻⁹ m

Length = 250 nm

From Ohm's Law ;

V = IR

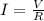`I = (V)/(R)` ---------- equation (1)

Also;

`R = \rho__0(L)/(A)`

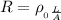
where:

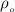`\rho __o` = resistivity of InSb = 4 × 10⁻¹³ Ω-m

L = length

A = area of cross section

Replacing our values in the above equation; we have:

`R = 4*10^(-13)*(250nm)/(150nm*250nm)`

`R = 4*10^(-13)*(1)/(150*10^(-9)m)`

`R = 2.6*10^(-6)` Ω

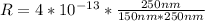
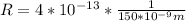
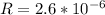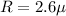`R = 2.6 \mu`Ω

From equation (1):

`I = (V)/(R)`

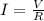
Therefore;

`I = (100*10^(-3))/(2.6*10^(-6))`

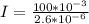
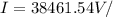`I =38461.54 V/`Ω

Since 1 V/Ω = 0.001 kilo ampere (KA)

Then;

`I =(38461.54 *0.001)KA`

`I =38.46KA`