Question

In testing a certain kind of truck tire over rugged terrain, it is found that 25% of the trucks fail to complete the test run without a blowout. Of the next 15 trucks tested, find the probability that less than 4 have blowouts.

Answer 1

The probability that of 15 trucks, less than 4 trucks have blowouts is 0.4613.

Explanation:

Let X = number of trucks having a blowout during the test run.

The probability of a truck having a blowout during the test run is, p = 0.25.

The sample of trucks selected is of size, n = 15.

The random variable X follows a Binomial distribution with parameters n = 15 and p = 0.25.

The probability mass function of X is:

`P(X=x)={15\choose x}0.25^(x)(1-0.25)^(15-x);\ x=0,1,2,3...`

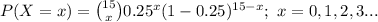
Compute the probability that less than 4 trucks have blowouts as follows:

P (X < 4) = P (X = 0) + P (X = 1) + P (X = 2) + P (X = 3)

`={15\choose 0}0.25^(0)(1-0.25)^(15-0)+{15\choose 1}0.25^(1)(1-0.25)^(15-1)\\+{15\choose 2}0.25^(2)(1-0.25)^(15-2)+{15\choose 3}0.25^(3)(1-0.25)^(15-3)\\=0.0134+0.0668+0.1559+0.2252\\=0.4613`

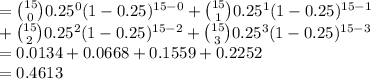
Thus, the probability that of 15 trucks, less than 4 trucks have blowouts is 0.4613.