Question

The radius of the path of an object in uniform circular motion is halved. The centripetal acceleration needed, given that

its speed remains the same, is
half as great
the same as before
twice as great as before
four times as great as before

Answer 1

twice as great as before

Step-by-step explanation:

recall that centripetal acceleration is given by
`a =(v^(2) )/(r)`

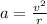
let the original radius of the circular motion = r₁ and the reduced radius be =r₂

acceleration for original radius, a₁ = v² / r₁ ==> v²=a₁ r₁

acceleration for reduced radius, a₂ = v² / r₂ ==> v²=a₂ r₂

given that the speed remains the same, we can equate the two expressions above

a₁ r₁ = a₂ r₂ (rearranging)

a₂ = (r₁ / r₂) a₁

we are given that r₂ = 1/2 r₁ (substitute this into equation)

a₂ = (r₁ / r₂) a₁

a₂ = [r₁ / (1/2)r₁ ] a₁

a₂ = [r₁ / (1/2)r₁ ] a₁

a₂ = 2a₁

hence we can see that the acceleration of the path with the smaller raidus has an acceleration that is twice the original