Answer:
The probability of 2 or more errors is 0.018.
Explanation:
Let X = number of typographical errors on a page of a certain magazine.
The expected number of errors is, λ = 0.2.
The random variable X follows a Poisson distribution with parameter λ = 0.2.
The probability mass function of X is:
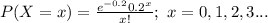
Compute the probability of 2 or more errors as follows:
P (X ≥ 2) = 1 - P (X < 2)
= 1 - P (X = 0) - P (X = 1)
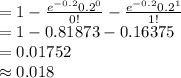
Thus, the probability of 2 or more errors is 0.018.