Answer:
99.73% of bags contain between 62 and 86 chips .
Explanation:
We are given that the number of chips in a bag is normally distributed with a mean of 74 and a standard deviation of 4.
Let X = percent of bags containing chips
So, X ~ N(
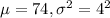 )
)
The standard normal z score distribution is given by;
Z =
 ~ N(0,1)
~ N(0,1)
So, percent of bags contain between 62 and 86 chips is given by;
P(62 < X < 86) = P(X < 86) - P(X <= 62)
P(X < 86) = P(
 <
<
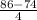 ) = P(Z < 3) = 0.99865 {using z table}
) = P(Z < 3) = 0.99865 {using z table}
P(X <= 62) = P(
 <=
<=
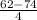 ) = P(Z <= -3) = 1 - P(Z < 3)= 1 - 0.99865 = 0.00135
) = P(Z <= -3) = 1 - P(Z < 3)= 1 - 0.99865 = 0.00135
So, P(62 < X < 86) = 0.99865 - 0.00135 = 0.9973 or 99.73%
Therefore, 99.73% of bags contain between 62 and 86 chips .