Question

Fowle Marketing Research, Inc., bases charges to a client on the assumption that telephone surveys can be completed in a mean time of 15 minutes or less. If a longer mean survey time is necessary, a premium rate is charged. Suppose a sample of 35 surveys shows a sample mean of 17 minutes. Use = 4 minutes. Is the premium rate justified? Click on the datafile logo to reference the data. a. Formulate the null and alternative hypotheses for this application. H 0: H a: b. Compute the value of the test statistic (to 2 decimals). c. What is the p-value (to 4 decimals)? d. Using = .01, is a premium rate justified for this client?

Answer 1

Test statistics = 2.96

P-value = 0.35%

Yes, a premium rate is justified for this client

Explanation:

We are given that a sample of 35 surveys shows a sample mean of 17 minutes and standard deviation of 4 minutes.

Also, we have to test that whether telephone surveys can be completed in a mean time of 15 minutes or less or not.

Null Hypothesis,
`H_0` :
`\mu` <= 15 minutes {means that the telephone surveys can be completed in a mean time of 15 minutes or less}

Alternate Hypothesis,
`H_1` :
`\mu` > 15 minutes {means that the telephone surveys take more than mean time of 15 minutes to get completed}

The test statistics we will use here is;

T.S. =
`(Xbar-\mu)/((s)/(√(n) ) )` ~
`t_n_-_1`

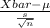where, X bar = sample mean = 17 minutes

s = sample standard deviation = 4 minutes

n = sample size = 35

So, Test statistics =
`(17-15)/((4)/(√(35) ) )` ~
`t_3_4`

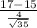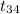
= 2.96

At 1% significance level, t table gives critical value of 2.441 at 34 degree of freedom. Since our test statistics is more than the critical value as 2.958 > 2.441 so we have sufficient evidence to reject null hypothesis as our test statistics will fall in the rejection region.

P-value is given by, P(
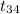`t_3_4` > 2.96) = 0.0035 or 0.35%

Here also, P-value is less than the significance level as 0.035% < 1% , so will reject null hypothesis.

Therefore, we conclude that the telephone surveys take more than mean time of 15 minutes to get completed and due to which a premium rate is charged and a premium rate is justified for this client.