Question

A Gallup Poll in July 2015 found that 26% of the 675 coffee drinkers in the sample said they were addicted to coffee. Gallup announced, "For results based on the sample of 675 coffee drinkers, one can say with 95% confidence that the maximum margin of sampling error is ±5 percentage points." (a) Confidence intervals for a percent follow the form estimate ± margin of error. Based on the information from Gallup, what is the 95% confidence interval for the percent of all coffee drinkers who would say they are addicted to coffee? (Enter your answers to the nearest percent.)

Answer 1

The 95% confidence interval for the percent of all coffee drinkers who would say they are addicted to coffee is between 21% and 31%.

Explanation:

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi(1-\pi))/(n)}`

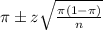
In which

z is the zscore that has a pvalue of
`1 - (\alpha)/(2)`.

The margin of error is:

`M = z\sqrt{(\pi(1-\pi))/(n)}`

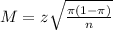
A confidence interval has two bounds, the lower and the upper

Lower bound:

`\pi - M`

Upper bound:

`\pi + M`

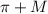
In this problem, we have that:

`\pi = 0.26, M = 0.05`

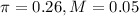
Lower bound:

`\pi - M = 0.26 - 0.05 = 0.21`

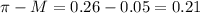
Upper bound:

`\pi + M = 0.26 + 0.05 = 0.31`

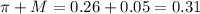
The 95% confidence interval for the percent of all coffee drinkers who would say they are addicted to coffee is between 21% and 31%.