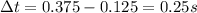Question

A weight is hung from a spring and set in motion so that it moves up and down continuously. The velocity v of the weight at any time t is given by the equation v = 1.5 cos(4πt) where v is measured in meters per second and t is measured in seconds. Find the maximum velocity of the weight and the amount of time it takes for the weight to move from its lowest position to its highest position.

Answer 1

0.25s

Explanation:

As v = 1.5 cos(4πt), v is maximum when cos(4πt) is maximum = 1. So the maximum velocity would be 1.5 m/s

Both the lowest and highest position occurs when its derivative is 0, aka v = 0 and has a change of direction

1.5 cos(4πt) = 0

cos(4πt) = 0

4πt = π/2 and 4πt = 3π/2

t = 1/8 = 0.125s and t = 3/8 = 0.375s

So the time it takes to travels from 1 point of time (0.125s) to another point of time (0.375) is

`\Delta t = 0.375 - 0.125 = 0.25s`