Question

When a certain string is under tension T, the speed of a wave in the string is v. What will be the speed of a wave in the string if the tension is increased to 2T without changing the mass or length of the string?

Answer 1

The wave speed would be increased by
`√(2)`

Step-by-step explanation:

As the formula for the wave speed of string v when subjected to tension T is:

`v = \sqrt{(T)/(\mu)}`

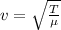
where μ is the string density (mass per length), which is constant for this case.

So when a string is subjected to tension 2T then

`(v_2)/(v) = (√(2T/\mu))/(√(T/\mu))`

`(v_2)/(v) = \sqrt{(2T)/(T)(\mu)/(\mu)} = √(2)`

`v_2 = √(2)v`

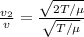
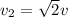
So the wave speed would be increased by
`√(2)`