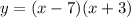The equation of the quadratic function is
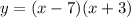
Step-by-step explanation:
The vertex form of the quadratic function is given by
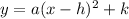
It is given that the quadratic function has a vertex at
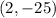
The vertex is represented by the coordinate
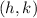
Hence, substituting
 in the vertex form, we get,
in the vertex form, we get,

Now, substituting the x - intercept
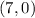 , we have,
, we have,
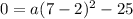
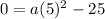
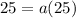
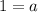
Thus, the value of a is 1.
Hence, substituting
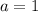 ,
,
 in the vertex form
in the vertex form
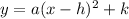 , we get,
, we get,
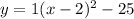
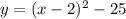
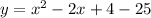
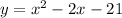
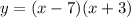
Thus, the equation of the quadratic function is