Question

Ming throws a stone off a bridge into a river below.

The stone's height (in meters above the water), 3 seconds after Ming threw it, is modeled by:

h(x) = -5(x - 1)2 + 45

How many seconds after being thrown will the stone reach its maximum height?

Please help!

Answer 1

45 meters.

Explanation:

its right, I tested it on khan

Answer 2

In 1 seconds after being thrown will the stone reach its maximum height.

Explanation:

Given : Ming throws a stone off a bridge into a river below. The stone's height (in meters above the water), 3 seconds after Ming threw it, is modeled by :
`h(x) = -5(x - 1)^2 + 45`.

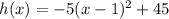To find : How many seconds after being thrown will the stone reach its maximum height?

Solution :

The maximum height is attain by getting the vertex of the model.

The vertex form of the quadratic equation or parabola is

`f (x) = a(x - h)^2+ k`

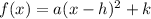
Where, (h,k) are the vertex.

On comparing with the given model,
`h(x) = -5(x - 1)^2 + 45`

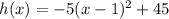
a=-5, h=1 and k=45

The vertex of the equation is (h,k)=(1,45).

Now the maximum height is 45 m and number of second is 1 sec.

Therefore, In 1 seconds after being thrown will the stone reach its maximum height.