Complete Question:
An isolated, charged conducting sphere of radius 11.0 cm creates an electric field of 4.90×10⁴ N/C at a distance 20.0 cm from its center. (a) What is its surface charge density? (b) What is its capacitance?
Answer:
(a) 1.47 x 10⁻⁶ C/m² or 1.47 μC/m²
(b) 12.07 x 10⁻¹² F or 12.07 pF
Step-by-step explanation:
The surface charge density, σ, of a surface (sphere, in this case) of area A which has a charge Q uniformly distributed on it is given by;
σ =
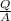 -----------------(i)
-----------------(i)
Also, the electric field, E, due to the charge Q, at a distance r, from the center of the sphere to another point on the sphere is given as;
E = k x
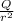 --------------(ii)
--------------(ii)
Where;
k = Coulomb's constant = 8.99 x 10⁹Nm²/C²
(a) i. First calculate the charge on the sphere as follows;
From the question;
r = 20.0cm = 0.20m
E = 4.90 x 10⁴ N/C
Substitute these values into equation (ii) as follows;
4.90 x 10⁴ = 8.99 x 10⁹ x

4.90 x 10⁴ = 8.99 x 10⁹ x
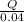
4.90 x 10⁴ = 224.75 x 10⁹ x Q
Q =

Q = 0.022 x 10⁻⁵
Q = 0.22 x 10⁻⁶ C
(a) ii. Also calculate the area A, of the sphere as follows;
A = 4π R²
Where;
R = radius of the sphere = 11.0cm = 0.11m
Substitute this value into equation above;
A = 4π (0.11)² [Take π = 3.142]
A = 4(3.142)(0.0121)
A = 0.15m²
(a) iii. Now calculate the surface charge density, of the sphere as follows;
Substitute the values of A and Q into equation (i) as follows;
σ =
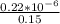
σ = 1.47 x 10⁻⁶C/m²
Therefore the surface charge density is 1.47 x 10⁻⁶C/m²
==============================================================
(b) The capacitance C, of an isolated charged sphere with radius R, is given by;
C = Aε₀ / R ----------------(iii)
Where;
R = 11.0cm = 0.11m
A = area of the sphere = 0.15m² [as calculated above]
ε₀ = permittivity of free space = 8.85 x 10⁻¹² C/Nm² [a known constant]
Substitute these values into equation (iii) as follows;
C = 0.15 x 8.85 x 10⁻¹² / 0.11
C = 12.07 x 10⁻¹²F
Therefore, the capacitance of the charged sphere is 12.07 x 10⁻¹²F