Question

The potential difference between a pair of oppositely charged parallel plates is 402 V. If the spacing between the plates is doubled without altering the charge on the plates, what is the new potential difference between the plates

Answer 1

The new potential difference between the plates is 804 V

Step-by-step explanation:

Capacitance of parallel plate capacitor is given by the relation:

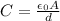`C=(\epsilon_(0)A )/(d)` ....(1)

Here A is area of each plate, D is distance between the two plates and ε₀ is vacuum permittivity constant.

The relation between charge on the plates and potential difference across the plates is:

Q = CV

Substitute the equation (1) in the above equation.

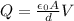`Q=(\epsilon_(0) A)/(d)V` .....(2)

The equation (2) represents the initial condition of the problem, when the plates are d distance apart.

According to the problem, now the distance between the plates get double but area of plates and charge on the plate remains same.

Rewrite equation (2) in terms of new potential difference V₀:

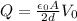`Q=(\epsilon_(0)A )/(2d) V_(0)` .....(3)

Equating equation (2) and (3).

`(\epsilon_(0)A )/(d) V=(\epsilon_(0)A )/(2d) V_(0)`

V₀ = 2V

Substitute 402 volts for V in the above equation.

V₀ = 2 x 402

V₀ = 804 volts