Question

Suppose you are repeating a classic study of starfish and their impact on the ecosystems of tide pools. Using historic data collected by you colleagues, you know that the mean number of starfish in the tide pool you are studying is 6.4. You are worried that a recent decline in starfish numbers in nearby pools may indicate a coming problem for your tide pool. If you estimate that the ecosystem of your tide pool will face significant negative consequences if the number of starfish in it drops below 3, calculate the probability of this occurring.

Answer 1

The probability of the number of starfish dropping below 3 is 0.0463.

Explanation:

Let X represent the number of starfish in the tide pool.

X follows a Poisson distribution with mean 6.4.

The formula for Poisson distribution is as follows.

`P(X=x) = e^(-6.4) * [6.4^(x) / x!] when x = 0, 1, ...`

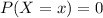`P(X = x) = 0` otherwise

We need to find the probability that the number of starfish in the tide pool drops below 3.

Therefore, the required probability is:

P(X < 3) = P(X = 0) + P(X = 1) + P(X=2)

`P(X < 3) = e^(-6.4) + (e^(-6.4)*6.4) + (e^(-6.4)*6.4^(2)/2)`

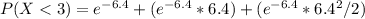
P(X < 3) = 0.00166 + 0.01063 + 0.03403

P (X < 3) = 0.0463