Question

Carbon absorbs energy at a wavelength of 150. nm. The total amount of energy emitted by a carbon sample is J. Calculate the number of carbon atoms present in the sample, assuming that each atom emits one photon.

Answer 1

The given question is incomplete. The complete question is as follows.

Carbon absorbs energy at a wavelength of 150 nm. The total amount of energy emitted by a carbon sample is
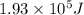`1.93 * 10^(5) J`. Calculate the number of carbon atoms present in the sample, assuming that each atom emits one photon.

Step-by-step explanation:

It is given that the energy at which C-atom absorbs energy is 150 nm. So, energy emitted by the carbon atom will have same wavelength at which C-atom absorbs the energy.

As we know that relation between energy and wavelength is as follows.

E =
`(hc)/(\lambda)`

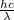
where, h = Planck's constant =
`6.624 * 10^(-34) J sec`

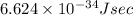
c = speed of light =
`3 * 10^(8) m/s`

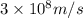
`\lambda` = 150 nm =
`150 * 10^(-9)`

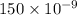
Therefore, energy of one carbon atom is calculated as follows.

E =
`(hc)/(\lambda)`

=
`(6.624 * 10^(-34) Js * 3 * 10^(8) m/s)/(150 * 10^(-9))`

=
`1.324 * 10^(-18) J`

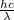
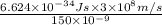
As the total energy emitted by the carbon sample is
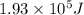`1.93 * 10^(5) J`. Let us assume that the number of C-atoms in the sample be x and it is calculated as follows.

`E_(total) = n * E_(1C-atom)`

n =
`(E_(total))/(E_(1C-atom))`

=
`(1.93 * 10^(5))/(1.324 * 10^(-18) J)`

=
`1.45 * 10^(23)`

Thus, we can conclude that number of carbon atoms present in the sample, are
`1.45 * 10^(23)`.