Answer:
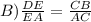
Explanation:
If ant two given triangles are SIMILAR, then they have equal corresponding angles and their corresponding sides are PROPORTIONAL.
For example: if Δ ABC ≈ Δ PQR, then
∠A = ∠P , ∠B = ∠Q and ∠C = ∠R
and
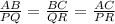
Now, here given: Δ ADE ≈ Δ ABC
Then by the SIMILAR postulate their corresponding angles are equal and their corresponding sides are Proportional.
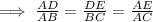 ............. (1)
............. (1)
Consider from above:
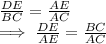 ............. (2)
............. (2)
Here, the given options are:
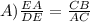 FALSE
FALSE
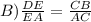 TRUE (from 2)
TRUE (from 2)
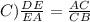 FALSE
FALSE
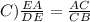 FALSE
FALSE