Question

A 4-kg toy car with a speed of 5 m/s collides head-on with a stationary 1-kg car. After the collision, the cars are locked together with a speed of 4 m/s. How much kinetic energy is lost in the collision?

Answer 1

Kinetic energy lost in collision is 10 J.

Step-by-step explanation:

Given,

Mass,
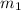`m_(1)` = 4 kg

Speed,
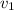`v_(1)` = 5 m/s

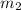`m_(2)` = 1 kg

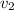`v_(2)` = 0

Speed after collision = 4 m/s

Kinetic energy lost, K×E = ?

During collision, momentum is conserved.

Before collision, the kinetic energy is

`(1)/(2) m1 (v1)^2 + (1)/(2) m2(v2)^2`

By plugging in the values we get,

`KE = (1)/(2) * 4 * (5)^2 + (1)/(2) * 1 * (0)^2\\\\KE = (1)/(2) * 4 * 25 + 0\\\\`

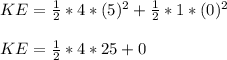
K×E = 50 J

Therefore, kinetic energy before collision is 50 J

Kinetic energy after collision:

`KE = (1)/(2) (4 + 1) * (4)^2 + KE(lost)`

`KE = 40J + KE(lost)`

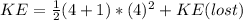
Since,

Initial Kinetic energy = Final kinetic energy

50 J = 40 J + K×E(lost)

K×E(lost) = 50 J - 40 J

K×E(lost) = 10 J

Therefore, kinetic energy lost in collision is 10 J.