Answer:
a) 81.85% of employees put between $9, 500 and $11,000 into the 401 k per year
b) 0.13% of employee put more than $11, 500 into the 401 k per year
c) 97.72% of employees put less than $11,000 into the 401k per year.
d) 97.72% of employees put more than $9,000 into the 401k per year
e) 2.15% of employees put between than $11,000 and $11, 500 into the 401k per year
f) An employee would need to put $10,640 into his or her 401 K to be in the upper 10% of investors
Explanation:
Problems of normally distributed samples are solved using the z-score formula.
In a set with mean
 and standard deviation
and standard deviation
 , the zscore of a measure X is given by:
, the zscore of a measure X is given by:

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.
In this problem, we have that:
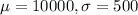
a. what proportion of employees put between $9, 500 and $11,000 into the 401 k per year
This is the pvalue of Z when X = 11000 subtracted by the pvalue of Z when X = 9500. So
X = 11000

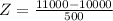
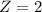
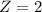 has a pvalue of 0.9772.
has a pvalue of 0.9772.
X = 9500

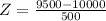
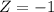
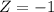 has a pvalue of 0.1587
has a pvalue of 0.1587
0.9772 - 0.1587 = 0.8185
81.85% of employees put between $9, 500 and $11,000 into the 401 k per year
b. What proportion of employee put more than $11, 500 into the 401 k per year?
This is 1 subtracted by the pvalue of Z when X = 11500. So

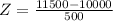
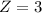
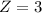 has a pvalue of 0.9987
has a pvalue of 0.9987
1 - 0.9987 = 0.0013
0.13% of employee put more than $11, 500 into the 401 k per year
c. What proportional of employees put less than $11,000 into the 401k per year?
This is the pvalue of Z when X = 11000. So

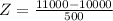
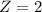
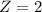 has a pvalue of 0.9772.
has a pvalue of 0.9772.
97.72% of employees put less than $11,000 into the 401k per year.
d. What proportional of employees put more than $9,000 into the 401k per year?
This is 1 subtracted by the pvalue of Z when X = 9000. So

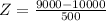

 has a pvalue of 0.0228.
has a pvalue of 0.0228.
1 - 0.0228 = 0.9772
97.72% of employees put more than $9,000 into the 401k per year
e. What proportional of employees put between than $11,000 and $11, 500 into the 401k per year?
This is the pvalue of Z when X = 11500 subtracted by the pvalue of Z when X = 11000. So
X = 11500

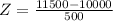
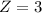
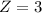 has a pvalue of 0.9987
has a pvalue of 0.9987
X = 11000

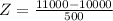
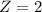
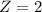 has a pvalue of 0.9772.
has a pvalue of 0.9772.
0.9987 - 0.0972 = 0.0215
2.15% of employees put between than $11,000 and $11, 500 into the 401k per year
f. How much would an employees need to put into his or her 401 K to be in the upper 10% of investors?
This is the value of Z when X has a pvalue of 1-0.1 = 0.9. So it is X when Z = 1.28.

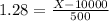
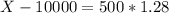

An employee would need to put $10,640 into his or her 401 K to be in the upper 10% of investors