Option C:
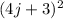 is the correct answer.
is the correct answer.
Step-by-step explanation:
The given expression is
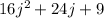
We need to factor the expression.
Let us rewrite the expression as

Also, we can rewrite the term
 as
as

Thus, we have,
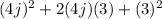
Hence, the equation is of the form,
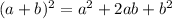
where
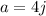 and
and
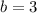
Hence, the factor of the expression can be written as
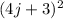
Thus, the factored expression is
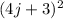
Therefore, Option C is the correct answer.