Question

The half-life of Carbon-14 is 5730 years. Suppose a fossil is found with 50% of Carbon-14 as compared to a living sample. How old is the fossil?

Answer 1

Answer: 5730 years

Step-by-step explanation:

Because it takes 5730 years for half of a sample of carbon-14 atoms to decay. It says that 50% of the carbon atoms have decayed so that means that 5730 years have elapsed for that fossil.

Answer 2

5732 years

Step-by-step explanation:

Radioactive decay can be determined by using the equation:

`N_t = N_0e^(- \lambda t)`

where;

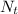`N_t` = number of decayed atoms at time (t)

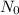`N_0` = initial number of decayed atoms

`\lambda` = decay constant

So, if we equate the natural log of the above; we have:

`In(N_t) = In(N_0)-\lambda t`

`\frac{In(N_t)} { In(N_0)}} = -\lambda t`

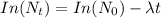
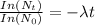
where;

`\lambda` =
`(0.693)/(t_(1/2))`

`\lambda` =
`(0.693)/(5730)`

`\lambda` =
`1.209*10^{-4`

`In((50)/(100)) =-(1.209 *10^(-4))*t`

`-0.693 = -(1.209*10^(-4))*t`

`t= (0.693)/(1.209*10^(-4))`

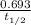
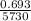
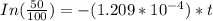
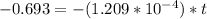
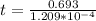
t = 5732.01 years

t = 5732 years.

Hence, the fossil is 5732 years old.