Question

The owner of a motel has 2900 m of fencing and wants to enclose a rectangular plot of land that borders a straight highway. If she does not fence the side along the highway, what is the largest area that can be enclosed?

Answer 1

Explanation:

Given that the owner of a motel has 2900 m of fencing and wants to enclose a rectangular plot of land that borders a straight highway.

Fencing is used for 2times length and 1 width if highway side is taken as width

So we have 2l+w = 2900

Or w = 2900-2l

Area of the rectangular region = lw

`A(l) = l(2900-2l) = 2900l-2l^2\\`

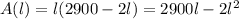
Use derivative test to find the maximum

`A'(l) = 2900-4l\\A`

So maximum when I derivative =0

i.e when
`l =(2900)/(4) =725`

Largest area = A(725)

=
`725(2900-2*725)\\= 1051250`

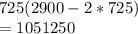
1051250 sqm is area maximum