Question

write a real word problem that can be represented by the equation ( 12 + 14 ) x h / 2 = 52. Then solve the problem.

Answer 1

Step-by-step explanation:

The area of a trapezoid is given by:

`A=((B_(1)+B_(2))h)/(2) \\ \\ \\ A;Area \\ \\ B_(1):First \ base \\ \\ B_(2)=Second \ base \\ \\ h:Heigth`

So given the equation:

`(( 12 + 14 )h)/(2) = 52`

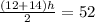
We can write a real problem as:

The bases of a trapezoid are
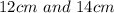`12cm \ and \ 14cm` and the area of it is
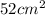`52cm^2`. What is the height of the trapezoid?

Solving:

`(( 12 + 14 )h)/(2) = 52 \\ \\ 26h=104 \\ \\ h=(104)/(26) \\ \\ h=4cm`

Finally, the height of the trapezoid is 4cm