Answer:
(a). The resultant of these forces is 1216.55 N.
(b). The direction of the resultant forces is 80.53°.
Step-by-step explanation:
Given that,
First force = 1200 N
Second force = 200 N
(a). We need to calculate the resultant of these forces
Using cosine law
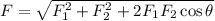
Put the value into the formula
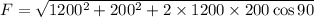
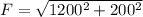

The resultant of these forces is 1216.55 N.
(b). We need to calculate the direction of the resultant forces
Using formula of direction
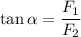
Put the value into the formula
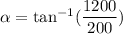
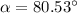
Hence, (a). The resultant of these forces is 1216.55 N.
(b). The direction of the resultant forces is 80.53°.