Since, you have not mentioned the statements, but I am solving the expression as well as verifying which anyways may be able to make you understand the concept.
Answer:
Both
 are the True solutions.
are the True solutions.
Explanation:
Considering the expression
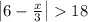
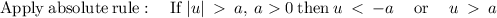

solving
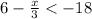
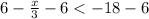
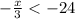
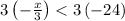
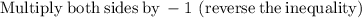
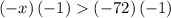

also solving
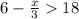
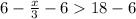

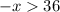
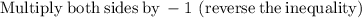
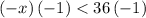
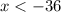

Verifying the solution:
Putting the value x < -36 in
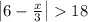
let suppose x = -37 which is < -36
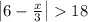



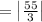
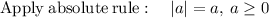
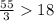
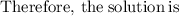
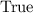
also putting the value x > 72
let suppose x = 73 which is > 72
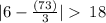
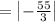
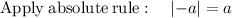
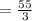
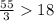
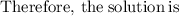
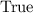
So, both
 are the True solutions.
are the True solutions.