Answer:
32.33% probability of having at least 3 erros in an hour.
Explanation:
In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

In which
x is the number of sucesses
e = 2.71828 is the Euler number
 is the mean in the given time interval.
is the mean in the given time interval.
The mean number of errors is 2 per hour.
This means that
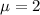
(a) What is the probability of having at least 3 errors in an hour?
Either you have 2 or less errors in an hour, or we have at least 3 errors. The sum of the probabilities of these events is decimal 1. So
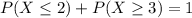
We want
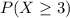
So
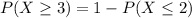
In which
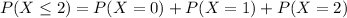

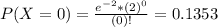
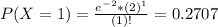
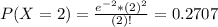
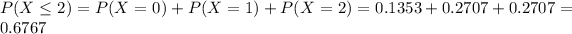
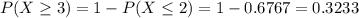
32.33% probability of having at least 3 erros in an hour.