Question

A tourist being chased by an angry bear is running in a straight line toward his car at a speed of 4.2 m/s. The car is a distance d away. The bear is 29 m behind the tourist and running at 6.0 m/s. The tourist reaches the car safely. What is the maximum possible value for d

Answer 1

Step-by-step explanation:

It is known that the relation between speed and distance is as follows.

velocity =
`(distance)/(time)`

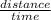
As it is given that velocity is 6 m/s and distance traveled by the bear is (d + 29). Therefore, time taken by the bear is calculated as follows.

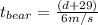`t_(bear) = ((d + 29))/(6 m/s)` ............. (1)

As the tourist is running in a car at a velocity of 4.2 m/s. Hence, time taken by the tourist is as follows.

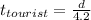`t_(tourist) = (d)/(4.2)` ............. (2)

Now, equation both equations (1) and (2) equal to each other we will calculate the value of d as follows.

`t_(bear) = t_(tourist)`

`((d + 29))/(6 m/s)` =
`(d)/(4.2)`

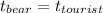
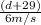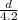
4.2d + 121.8 = 6d

d =
`(121.8)/(1.8)`

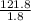
= 67.66

Thus, we can conclude that the maximum possible value for d is 67.66.