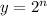Question

James folds a piece of paper in half several times,each time unfolding the paper to count how many equal parts he sees. After folding the paper about six times, ti becomes too difficult to fold it again,but he is curious how many parts the paper would be broken into if he could continue to fold it. He decides to employ the modeling cycle to predict how many parts the paper would be folded into if he were able to fold it 11 times.

Answer 1

There will be total 2048 parts of the given paper if James if able to fold the paper eleven times.

The needed function is
`y = 2 ^n`

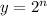
Explanation:

Let us assume the piece of paper James decides to fold is a SQUARE.

Now, let us assume:

n : the number of times the paper is folded.

y : The number of parts obtained after folds.

Now, if the paper if folded ONCE ⇒ n = 1

Also, when the pap er is folded once, the parts obtained are TWO equal parts.

⇒ for n = 1 , y = 2 ..... (1)

Similarly, if the paper if folded TWICE ⇒ n = 2

Also, when the paper is folded twice, the parts obtained are FOUR equal parts.

⇒ for n = 2 , y = 4 ..... (2)

⇒
`y = 2^2 = 2^n`

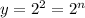
Continuing the same way, if the paper is folded SEVEN times ⇒ n = 7

So,
`y = 2^ n = 2^7 = 128`

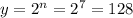
⇒ There are total 128 equal parts.

Lastly, if the paper is folded ELEVEN times ⇒ n = 11

So,
`y = 2^ n = 2^(11) = 2048`

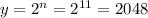
⇒ There are total 2048 equal parts.

Hence, there will be total 2048 parts of the given paper if James if able to fold the paper eleven times.

And the needed function is
`y = 2 ^n`