Question

A laboratory dish, 20 cm in diameter, is half filled with water. One at a time, 0.49 μL drops of oil from a micropipette are dropped onto the surface of the water, where they spread out into a uniform thin film. After the first drop is added, the intensity of 640 nm light reflected from the surface is very low. As more drops are added, the reflected intensity increases, then decreases again to a minimum after a total of 13 drops have been added. What is the index of refraction of the oil?

Answer 1

Step-by-step explanation:

Formula for path difference is as follows.

x = 2tn

and, refractive index (n) =
`(\lambda)/(2t)`

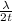
Thickness is calculated as follows.

Thickness (t) =
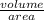
`(volume)/(area)`

Area =
`\pi r^(2)`

=
`\pi * ((d)/(2))^(2)`

=
`(0.49 * 10^(-6))/(3.14 * 0.01 m)`

=
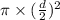
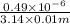
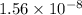`1.56 * 10^(-8)` m

Now, the refractive index will be calculated as follows.

For drop, n =
`(\lambda)/(2t)`

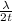
For B drop, n =
`(\lambda)/(26t)`

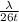
So, n =
`(640 * 10^(-9))/(26 * 1.56 * 10^(-8))`

=
`(640 * 10^(-9))/(40.56 * 10^(-8))`

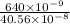
= 1.5

Thus, we can conclude that index of refraction of the oil is 1.5.