Answer:
The distance between M(7, 8) and N(-3,-8) is 18.87.
Step-by-step Step-by-step explanation:
Solution :
Here's the required formula to find distance:
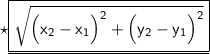
Substituting all the given values in the formula to find distance :
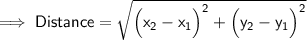

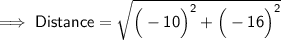
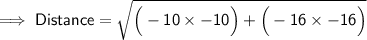
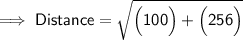
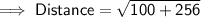

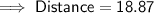
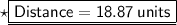
Hence, the distance between M(7, 8) and N(-3,-8) is 18.87.
