Answer:
a) Gold = $1,380; Silver = $1,020
b) Gold = $1,300; Silver = $980
Step-by-step explanation:
a) At first, with Qg = 60 and Qs = 270, the equilibrium prices for gold and silver are found by solving the following linear system:
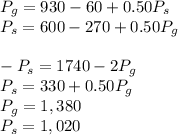
Equilibrium price of gold is $1,380 and the price of silver is $1,020.
b) If the supply of gold increases to 120, since the goods are substitutes, there will be an increase in overall supply and the equilibrium price of gold and silver will decrease as follows:
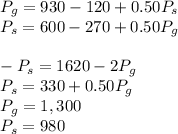
Equilibrium price of gold is $1,300 and the price of silver is $980.