Answer:
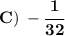
Explanation:
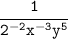
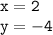
~
Substitute ''x'' with '2' & 'y' with "-4":-
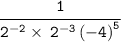
First let's solve
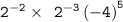
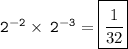
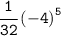
Calculate exponents:- -4^5= -1024
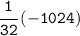
Remove parentheses, apply rule:-
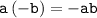
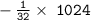
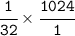
Apply fraction rule:-
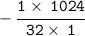
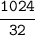
Divide numbers:-

Now that we're done factoring the denominator let's bring down the numerator which is ' 1 ':-
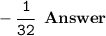
☆-------☆-------☆-------☆