Question

crate sits on a horizontal floor where the coefficient of static friction between the crate and the floor is 0.50. A 20-N force is applied to the crate acting to the right. What is the resulting static friction force acting on the crate

Answer 1

The resulting static friction force acting on the crate is 10 N.

Step-by-step explanation:

Given that,

Coefficient of static friction = 0.50

Normal force = 20 N

We need to calculate the resulting static friction force acting on the crate

Using formula of static friction force

`f_(s)=\mu_(s)N`

Where, N = normal force

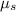`\mu_(s)` = Coefficient of static friction

Put the value into the formula

`f_(s)=0.50*20`

`f_(s)=10\ N`

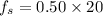
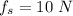
Hence, The resulting static friction force acting on the crate is 10 N.