Answer:
24m
Step-by-step explanation:
Consider one of the equations of motion as follows;
s = ut +
 at² ----------------------------(i)
at² ----------------------------(i)
Where;
s = vertical/horizontal displacement of the body in motion
u = initial vertical/horizontal displacement of the body
t = time taken for the displacement
a = vertical/horizontal acceleration of the body.
Now, since the particle being considered moves in an xy-coordinate system, then equation (i) above can be resolved into the x (horizontal) and y (vertical) components as follows;
Horizontal (x-coordinate) component
 =
=
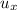 t +
t +

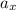 t² ------------------(ii)
t² ------------------(ii)
Where;
 = horizontal displacement (x-coordinate) of the particle in motion
= horizontal displacement (x-coordinate) of the particle in motion
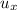 = initial horizontal displacement of the particle
= initial horizontal displacement of the particle
t = time taken for the displacement
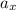 = horizontal (x-direction) acceleration of the body.
= horizontal (x-direction) acceleration of the body.
Vertical (y-coordinate) component
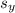 =
=
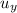 t +
t +

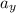 t² -------------------(iii)
t² -------------------(iii)
Where;
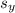 = vertical displacement (y-coordinate) of the particle in motion
= vertical displacement (y-coordinate) of the particle in motion
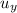 = initial vertical displacement of the particle
= initial vertical displacement of the particle
t = time taken for the displacement
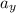 = vertical (y-direction) acceleration of the body.
= vertical (y-direction) acceleration of the body.
(A) Now, using equation (iii), from the question;
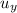 = 0 [since the particle starts from rest, initial velocity is zero]
= 0 [since the particle starts from rest, initial velocity is zero]
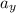 = 1m/s² [acceleration in the y-direction]
= 1m/s² [acceleration in the y-direction]
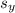 = 12m [y-coordinate value]
= 12m [y-coordinate value]
Substitute these values into equation (iii) as follows;
12 = 0 t +
 (1) t²
(1) t²
12 =
 t² [Multiply through by 2]
t² [Multiply through by 2]
24 = t² [Solve for t]
t =
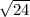 seconds
seconds
(B) Also, using equation (ii), from the question;
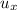 = 0 [since the particle starts from rest, initial velocity is zero]
= 0 [since the particle starts from rest, initial velocity is zero]
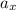 = 2m/s² [acceleration in the x-direction]
= 2m/s² [acceleration in the x-direction]
 = ? [x-coordinate value]
= ? [x-coordinate value]
Substitute these values into equation (ii) as follows;
 = 0 t +
= 0 t +
 (2) t²
(2) t²
 = t² -------------------(iv)
= t² -------------------(iv)
But t =
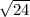 seconds as calculated above, substitute this value into equation (iv)
seconds as calculated above, substitute this value into equation (iv)
 = (
= (
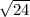 )² [Solve for
)² [Solve for
 ]
]
 = 24
= 24
Therefore, the x-coordinate of the particle when the y-coordinate is 12m is 24m