Question

Jacob and Sophia are playing with a merry-go-round on a playground.The merry-go-round can be modeled as a flat disk with massM= 223.0kg and radiusR= 3.3 m that freely rotates horizontally without frictionabout its center axis. When the merry-go-round is already spinning at23 rotations per minute, Jacob exerts a constant forceFJ= 12.3 Ntangent to the outer edge of the merry go round in the direction of themerry-go-round’s rotation. At the same time Sophia puts her foot ata distancer= 3.1 m from the center, which exerts a constant force offrictionFS= 21.2 N. How long will it take for the merry-go-round tocome to a stop?

Answer 1

116 s

Step-by-step explanation:

We can convert the 23 rotation per minute to radian per second knowing each rotation is 2π and each minute has 60 seconds.

`\omega_0 = 2\pi*23/60 = 2.41 rad/s`

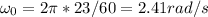
The torque generated by Jacob at the outer rim in the direction of motion

`T_J = F_JR_J = 12.3 * 3.3 = 40.59 Nm`

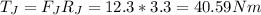
The torque generated by Sophia at r = 3.1 m that hampers the motion is

`T_S = F_Sr = 21.2*3.1 = 65.72 Nm`

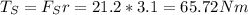
The net torque is

`T = T_S - T_J = 65.72 - 40.59 = 25.13 Nm`

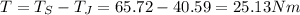
The moment of inertia of the solid disk merry-go-round is:

`I = mR^2/2`

Where m = 223 kg is the disk mass and R = 3.3 m is the radius of the disk.

`I = 223*3.3^2/2 = 1214.235 kgm^2`

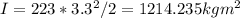
So the angular deceleration is

`\alpha = T / I = 25.13 / 1214.235 = 0.0207 rad/s^2`

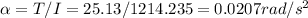
If the initial angular speed is 2.41, the time it'd take to decelerate to rest is

`t = \Delta \omega / \alpha = (0 - 2.41)/(-0.0207) = 116 s`