The new length will be 7.15 cm. Option C.
Explanation:
Given,
The length of the floor is 33 feet.
The length of the floor should be increased by 30%.
Also, 1 cm = 6 ft
To find the scale of this change.
Now,
The length will be increased by = 30×33% ft
= 30×
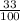 = 9.9 ft
= 9.9 ft
Again,
6 ft = 1 cm
1 ft =

9.9 ft =
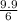 cm = 1.65 cm
cm = 1.65 cm
And
33 ft =
 cm = 5.5 cm
cm = 5.5 cm
Hence,
The new length will be = 5.5+1.65 cm = 7.15 cm