Question

Phoebe's insulated foam cup is filled with 149.0 g of coffee (mostly water - c = 4.2 J/g·°C) that is too hot to drink, so she adds 11.0 g of milk at 9.0 °C. If the coffee has an initial temperature of 65.0 °C and the specific heat of milk is 3.8 J/g·°C, how hot is the coffee after the milk is added? (Assume that no heat leaks out through the cup.)

Answer 1

`61.5^(\circ)C`

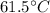
Step-by-step explanation:

When the cold milk is added into the hot coffee, heat is transferred from the coffee (higher temperature) to the milk (lower temperature), until the two substances are at the same temperature (thermal equilibrium).

Therefore, the heat given off by the coffee is equal to the heat absorbed by the milk; so, we can write:

`Q_c=Q_m\\m_c C_c (T_c-T_e)=m_m C_m (T_e-T_m)`

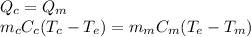
where:

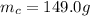`m_c=149.0 g` is the mass of coffee

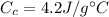`C_c=4.2 J/g^(\circ)C` is the specific heat capacity of coffee

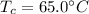`T_c=65.0^(\circ)C` is the initial temperature of the coffee

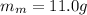`m_m = 11.0 g` is the mass of milk

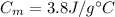`C_m=3.8J/g^(\circ)C`is the specific heat capacity of milk

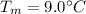`T_m=9.0^(\circ)C` is the initial temperature of milk

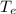`T_e` is the temperature at equilibrium

Solving for
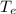`T_e`, we find the final temperature of the coffee at equilibrium:

`T_e=(m_c C_c T_c+m_m C_m T_m)/(m_c C_c + m_m C_m)=((149)(4.2)(65)+(11)(3.8)(9))/((149)(4.2)+(11)(3.8))=61.5^(\circ)C`