Answer:
C)
Minimum of t =
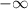
Maximum of t =
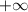
Minimum of h(t) =
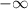
Maximum of h(t) = +20
D)
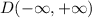
![R(-\infty,+20]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/p9b35b58vm0svj7ahosiv8z71z66if3soo.png)
Explanation:
C)
Here in this problem, we are given the function, which is
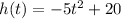
We observe immediately the following:
- The function has no limitations on the value of t - in fact, it contains no square roots, no logarithms, and no fractions; therefore, every value of x is acceptable in this function. This means that it has the variable t has no minimum or maximum values.
- On the other hand, h(t) cannot take any value. In fact, we notice that this is a quadratic function with the second-degree term with a negative coefficient: this means that it is a downward parabola. So, it has no downward limit (it goes to
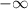 ), but it has a maximum, which corresponds to the vertex of the parabola.
), but it has a maximum, which corresponds to the vertex of the parabola.
The x-coordinate of the parabola is given by

because the coefficient of the 1st-degree term, b, is zero. So, the y-coordinate of the vertex is
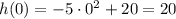
So, the maximum of h(t) is 20. Therefore we have:
Minimum of t =
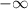
Maximum of t =
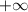
Minimum of h(t) =
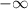
Maximum of h(t) = +20
D)
The domain of a function is defined as the set of values of the independent function x for which the function has a valid value.
Therefore in this case, the domain of h(t) is the set of all values allowed for t: so, from part C, we can say that the domain is
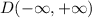
So, all real values.
The range of a function instead is the set of all values of the dependent variables, y.
So in this case, the range of h(t) is the set of all values that h(t) can take.
From part C, we know therefore that the range is
![R(-\infty,+20]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/p9b35b58vm0svj7ahosiv8z71z66if3soo.png)
Where +20 has the ] instead of ) because it is also an allowed value.