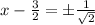Answer:

Explanation:
we have
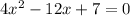
step 1
Group terms that contain the same variable, and move the constant to the opposite side of the equation
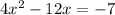
step 2
factor the leading coefficient

step 3
Complete the square. Remember to balance the equation by adding the same constants to each side
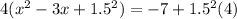

simplify

step 4
Rewrite as perfect squares
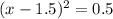
step 5
take square root both sides
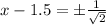
Convert 1.5 in a fraction number