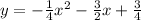Question

What is the standard form given the vertex (-3,3) and the focus point (-3,2)

Answer 1

`y=-(1)/(4)x^2-(3)/(2)x+(3)/(4)`

Explanation:

The standard form of a parabola is written as

`y=ax^2+bx+c`

where a, b and c are the coefficients of the second-degree, first degree and zero-degree terms.

The coordinates of the vertex of a parabola is given by:

`x_b = -(b)/(2a)`

`y_b=c-(b^2)/(4a)`

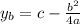
The coordinates of the focus instead are given by

`x_f=-(b)/(2a)`

`y_f=y_b+(1)/(4a)`

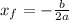
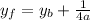
In this problem, we know the coordinates of the vertex and of the focus point:

Vertex: (-3,3)

Focus point: (-3,2)

So we have:

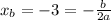`x_b=-3=-(b)/(2a)` (1)

`y_b=3=c-(b^2)/(4a)` (2)

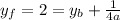`y_f=2=y_b+(1)/(4a)` (3)

From eq.(1) we get

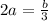`2a=(b)/(3)` (4)

Substituting into (2),

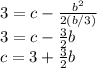`3=c-(b^2)/(2(b/3))\\3=c-(3)/(2)b\\c=3+(3)/(2)b`(5)

Now rewriting eq.(3) as

`2=y_b+(1)/(4a)\\2=(c-(3)/(2)b)+(1)/(4a)`

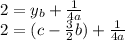
And substituting (4) and (5) into this, we can find b:

`2=((3+(3)/(2)b)-(3)/(2)b)+(1)/(2(b/3))\\2=3+(3)/(2b)\\-1=(3)/(2b)\\b=-(3)/(2)`

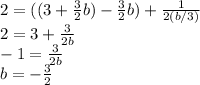
Then we can find a and c:

`2a=(b)/(3)\\a=(b)/(6)=(-3/2)/(6)=-(1)/(4)`

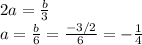
And

`c=3+(3)/(2)b=3+(3)/(2)(-(3)/(2))=3-(9)/(4)=(3)/(4)`

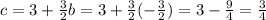
So the parabola is

`y=-(1)/(4)x^2-(3)/(2)x+(3)/(4)`