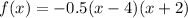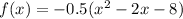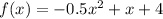Answer:
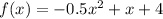
Explanation:
we know that
A quadratic function in factored form is equal to
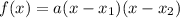
where
a is the leading coefficient
x_1 and x_2 are the zeroes of the function
substitute the given values
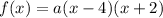
Find the value of a
Remember that
For x=0 ----> f(0)=4
substitute
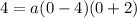
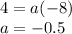
so