The equation of the circle is = (x +5)² + (y -8)² = 10.
Explanation:
Step 1; The given coordinates are taken as (x1, y1) and (x2, y2). So
(x1, y1) = (-4, 11) and (x2, y2) = (-6, 5).
The midpoint of the circle, (h, k) = (
,
).
For the given circle, (h, k) = (
,
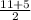) = (-5, 8)
Step 2; To find the distances between the midpoint (-5, 8) and another point on the circle (-6, 5), we use the formula
Distance = √ (x2 - x1)² + (y2 - y1)².
Distance between (-4, 11) and (-6, 5) is,
Distance = √ (-6 - (-4))² + (5 - 11)² = √ 4 + 36 = √40 = Radius of the cirlce (r).
Step 3; The equation of a circle is given by (x -h)² + (y -k)² = r².
So the equation is (x +5)² (y -8)² = (√10)² = 10.