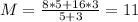Answer:
a) For this case we can use the definition of weighted average given by:

And if we replace the values given we have:

b)
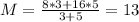
c)
Explanation:
Assuming the following question: "One sample has a mean of M=8 and a second sample has a mean of M=16 . The two samples are combined into a single set of scores.
a) What is the mean for the combined set if both of the original samples have n=4 scores "
For this case we can use the definition of weighted average given by:

And if we replace the values given we have:

b) what is the mean for the combined set if the first sample has n=3 and the second sample has n=5
Using the definition we have:
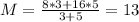
c) what is the mean for the combined set if the first sample has n=5 and the second sample has n=3
Using the definition we have: