Answer:
a) 0.149
b) (0.135,0.163)
c) (0.134,0.164)
Explanation:
We are given the following in the question:
a) Sample size, n = 2336
Number of people that have at least one tattoo, x = 349
Point Estimate:
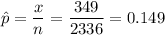
b) 95% confidence interval:
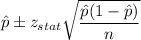
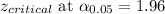
Putting the values, we get:

c) 98% confidence interval
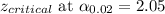
Putting the values, we get:

d) With increase in confidence level, the width of the confidence interval increases.