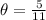Question

find the radian measure of the centeral angle of a circle of radius r and arc length of s. a. R=22 ft and s=10ft

Answer 1

`\theta=0.45rad`

Explanation:

The radian measure of the centeral angle of a circle of radius r and arc length of s, can be calculated using the relation:
`s=r\theta`

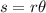
The radius is given as
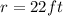`r=22ft` and the arc length is given as:
`s=10ft`

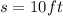
Let us substitute the values into the formula and solve for
`\theta`.

This implies that:

`10=22\theta`

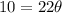
We divide both sides by 22

`(10)/(22)=\theta`

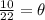
Therefore
`\theta=(5)/(11)`