Answer:
3.70% probability she allows more than one goal
Explanation:
In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

In which
x is the number of sucesses
e = 2.71828 is the Euler number
 is the mean in the given time interval.
is the mean in the given time interval.
X~Pois(0.3)
This means that
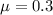
In her next match, what is the probability she allows more than one goal?
Either she allows at most one goal, or she allows more than one goal. The sum of the probabilities of these events is decimal 1. So
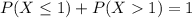
We want
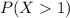 . So
. So
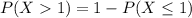
In which
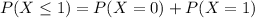

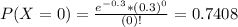
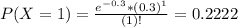

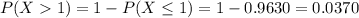
3.70% probability she allows more than one goal