Question

Two ice climbers, Shirly and Tim, are walking up a steep ice slope with an incline angle of 40 degrees . They are simply walking up it with ice spikes. The ice spikes give each of them a coefficient of kinetic friction of 0.7 as long as they are standing. The climbers are attached by a long length of rope. Tim trips, falls, and begins sliding down the slope. Unfortunately, there is a shear drop off not far behind them. Tim goes over the edge.1 ) When the rope goes taut, if both climbers have masses of 60 kg for Shirly and 80 kg for Tim, what is Shirly’s acceleration as she is pulled towards the cliff (she keeps her ice spikes dug into the ground)?

2) While sliding, Shirly builds up a speed of 5 m/s before pulling out her climbing axe and burying it into the hillside! This increases her total coefficient of kinetic friction 2.6 . What is her acceleration now?

3) How far down the slope does she slide after using the axe?

4) Shirly manages to stop their decent. While stopped, with the axe in the ice, her coefficient of static friction is 2.8 . What is the maximum acceleration ice Tim can climb up the rope, without causing them to slide again?

Answer 1

1) a = 14.125 m/s^2

2) a = -0.1529 m/s^2

3) s = 81.753 meters

4) a = 1.242 m/s^2

Step-by-step explanation:

Let's first express the normal force that Shirly exerts on the ground.

This is:

F = m * g * Cos(40°)

F = 60 * 9.81 * Cos(40°)

F = 450.89 N

The force pulling her down the slope will be:

F = Weight of Tim + Component of Shirly's weight acting down the incline

F = (80 * 9.81) + (60 * 9.81 * Sin (40°) )

F = 1163.14 N

Let's also find the frictional force on shirly as she slides down:

Friction = u * R

Friction = 0.7 * 450.89

Friction = 315.623 N

1) The acceleration of shirly can be given by using the resultant force as follows:

F = m * a

(1163.14 - 315.623) = 60 * a

a = 14.125 m/s^2

2) First we calculate the new friction, and use the force balance to solve for the acceleration again like above.

New friction = u * R

New friction = 2.6 * 450.89

New friction = 1172.314 N

F = m * a

(1163.14 - 1172.314) = 60 * a

a = -0.1529 m/s^2

3) Since we know her initial speed and acceleration, and by setting the final speed equal to 0, we get the following equation of motion:

`v^2 - u^2 = 2*a*s`

`0^2 - 5^2 = 2*(-0.1529)*s`

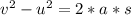
s = 81.753 meters

4) Lets start by calculating the new friction again. This is:

New friction = u * R

New friction = 2.8 * 450.89

New friction = 1262.49 N

Total force = 1163.14 - 1262.49 = -99.35 N

To find the acceleration for Tim we use the following formula:

F = m * a

99.35 = 80 * a

a = 1.242 m/s^2