2. TU, UV and TV are mid-segments
3. Definition of mid-segment
4. Division property of equality
6. SSS similarity theorem
Solution:
Step 1: Given
T is the midpoint of QR.
U is the midpoint of QS.
V is the midpoint of RS.
Step 2: Mid-segments connects midpoints of opposite sides.
TU, UV and TV are mid-segments.
Step 3: By definition of mid-segment
A triangle mid-segment is parallel to the third side of the triangle and is half of the length of the third side.
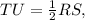
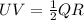 and
and

Step 4: By division property of equality
Divide first segment by RS, second segment by QR and third segment by SQ.

Step 5: By transitive property

Step 6: From the above steps, the sides of the triangle are congruent.
By SSS similarity theorem,
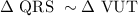
Hence proved.